$
\newcommand{\classe}{\square}
\newcommand{\hierarchie}{\square \raise 3pt{\moveleft 3pt \square}}
\DeclareMathOperator{\ou}{\vdots}
\DeclareMathOperator{\correlation}{\ou}
\newcommand{\analyse}{\space :: \space}
\newcommand{\relation}{{\rm R}}
\DeclareMathOperator{\et}{R}
\newcommand{\fonction}{\varphi}
\newcommand{\inclut}{\rhd}
\newcommand{\entre}{\lhd}
\newcommand{\mutation}{¡}
\newcommand{\semiotique}{\gamma \deg g \deg}
\newcommand{\plan}{_\star g\deg}
\newcommand{\plancontenu}{\gamma \deg}
\newcommand{\planexpression}{g \deg}
\newcommand{\planinterne}{\interne \plan}
\newcommand{\planexterne}{\externe \plan}
\newcommand{\plandenotatif}{\externe \planexpression}
\newcommand{\planconnotatif}{\externe \plancontenu}
\newcommand{\plansemiologique}{_{2\star} g \deg}
\newcommand{\planmetasemiologique}{_{3\star} g \deg}
\newcommand{\plansemiologiqueinterne}{\interne \plansemiologique}
\newcommand{\plansemiologiqueexterne}{\externe \plansemiologique}
\newcommand{\planmetasemiologiqueinterne}{\interne \planmetasemiologique}
\newcommand{\planmetasemiologiqueexterne}{\externe \planmetasemiologique}
\newcommand{\uniteextrinseque}{^\frown}
\newcommand{\categorieextrinseque}[1]{)#1(}
\newcommand{\glossematie}{\uniteextrinseque}
\newcommand{\intrinseque}{^\asymp}
\newcommand{\plerematie}{\gamma\glossematie}
\newcommand{\plerematieintrinseque}{\gamma\intrinseque}
\newcommand{\plerematiepour}{\exists}
\newcommand{\manifestante}{\land}
\newcommand{\manifestee}{\lor}
\newcommand{\chaine}{^N}
\newcommand{\chainecontenu}{\gamma^N}
\newcommand{\chaineexpression}{g^N}
\newcommand{\categorie}[1]{\{#1\}}
\newcommand{\cat}{\categorie}
\newcommand{\unite}{^{n}}
\newcommand{\Unite}[1]{^{#1}}
\newcommand{\unitede}[1]{{#1}^n}
\newcommand{\unitedepour}[2]{{#1}^{#2}}
\newcommand{\deg}{^{\circ}}
\newcommand{\cata}{\{ \ou \alpha \}}
\newcommand{\catA}{\{ \ou A\}}
\newcommand{\catb}{\{ \ou \beta \}}
\newcommand{\catB}{\{ \ou B \}}
\newcommand{\catg}{\{ \ou \gamma \}}
\newcommand{\catG}{\{ \ou \Gamma \}}
\newcommand{\catbd}{\{ \ou \beta _{2} \}}
\newcommand{\catBd}{\{ \ou B _{2} \}}
\newcommand{\catgd}{\{ \ou \gamma _{2} \}}
\newcommand{\catGd}{\{ \ou \Gamma _{2} \}}
\newcommand{\catc}{\catg}
\newcommand{\catcd}{\catgd}
\newcommand{\catC}{\catG}
\newcommand{\catCd}{\catGd}
\newcommand{\solidarite}{\sim}
\newcommand{\Solidarite}{\et \ou \beta}
\newcommand{\combinaison}{-}
\newcommand{\selection}{\rightarrow}
\newcommand{\Selection}{\et \ou \gamma}
\newcommand{\Combinaison}{\et \ou B}
\newcommand{\selectionne}{\leftarrow}
\newcommand{\selectionnant}{\rightarrow}
\newcommand{\selectionnenant}{\rightleftarrows}
\newcommand{\selectionneetnant}{\selectionne \selectionnant}
\newcommand{\selectionneselectionnant}{\rightleftarrows}
\newcommand{\interdependance}{\leftrightarrow}
\newcommand{\Interdependance}{\varphi \ou \beta}
\newcommand{\Constellation}{\varphi \ou B}
\newcommand{\Determination}{\varphi \ou \gamma}
\newcommand{\variete}{\solidarite \variante}
\newcommand{\variation}{\combinaison \variante}
\newcommand{\casea}{\ou a}
\newcommand{\caseb}{\ou b}
\newcommand{\casec}{\ou c}
\newcommand{\caseab}{\ou ab}
\newcommand{\caseac}{\ou ac}
\newcommand{\casebc}{\ou bc}
\newcommand{\caseabc}{\ou abc}
\newcommand{\participanta}{\ou \alpha}
\newcommand{\participantA}{\ou A}
\newcommand{\participantb}{\ou \beta}
\newcommand{\participantB}{\ou B}
\newcommand{\participantg}{\ou \gamma}
\newcommand{\participantG}{\ou \Gamma}
\newcommand{\participantbd}{\ou \beta_2}
\newcommand{\participantBd}{\ou B_2}
\newcommand{\participantgd}{\ou \gamma_2}
\newcommand{\participantGd}{{\ou \Gamma_2}}
\newcommand{\participantbdeux}{\ou \beta_2}
\newcommand{\participantBdeux}{\ou B_2}
\newcommand{\participantgdeux}{\ou \gamma_2}
\newcommand{\participantGdeux}{\ou \Gamma_2}
\newcommand{\participantGb}{\ou \Gamma_{\beta}}
\newcommand{\participantGB}{\ou \Gamma_{B}}
\newcommand{\participantGg}{\ou \Gamma_{\gamma}}
\newcommand{\zonea}{\ou (\alpha)}
\newcommand{\zoneb}{\ou (\beta)}
\newcommand{\zoneg}{\ou (\gamma)}
\newcommand{\contraire}{a \ou b}
\newcommand{\contradictoire}{ab \ou c}
\newcommand{\correlationsimple}{a \ou b(c)}
\newcommand{\unitecomplexe}{^{\gt_1}}
\newcommand{\duplexe}{^2}
\newcommand{\Duplexe}[1]{{#1}^2}
\newcommand{\triplexe}{^3}
\newcommand{\Triplexe}[1]{{#1}^3}
\newcommand{\simplexe}{^1}
\newcommand{\quadruplexe}{^4}
\newcommand{\Quadruplexe}[1]{{#1}^4}
\newcommand{\complementarite}{\unicode{9537}}
\newcommand{\complementaritebis}{\unicode{620}}
\newcommand{\autonomie}{\unicode{9536}}
\newcommand{\etablissante}{\unicode{8634}}
\newcommand{\etablie}{\unicode{8635}}
\newcommand{\suspension}{^-}
\newcommand{\superposition}{/}
\newcommand{\defectiver}{\downarrow}
\newcommand{\defectivee}{\uparrow}
\newcommand{\dominante}{\in}
\newcommand{\dominee}{\ni}
\newcommand{\facultatif}[1]{(#1)}
\newcommand{\latent}[1]{^{#1}}
\newcommand{\actualisee}[1]{\{#1\}}
\newcommand{\intensifa}{\participanta}
\newcommand{\intensifb}{\participantb}
\newcommand{\intensifg}{\participantg}
\newcommand{\unite}{{}^n}
\newcommand{\constante}{a}
\newcommand{\variable}{x}
\newcommand{\fonctif}{p}
\newcommand{\specifiant}{\vdash}
\newcommand{\specifie}{\dashv}
\newcommand{\realisee}{X}
\newcommand{\pre}{?}
\newcommand{\conformite}{\Arrowvert}
\newcommand{\cohesionparadigmatiquebis}{\moveright 1pt \infty \moveleft 4pt |}
\newcommand{\cohesionparadigmatique}{ȸ}
\newcommand{\cohesionsyntagmatique}{\moveright 6pt {\diagup \moveleft 4pt \diagup} \moveleft 6pt {\diagdown \moveleft 4pt \diagdown } }
\newcommand{\catalysee}{\geq}
\newcommand{\connectif}{_\star X}
\newcommand{\indicateur}{\moveright 2pt {\lower 2pt \circ} \moveleft 5pt \nearrow}
\newcommand{\signal}{\unicode{9808}}
\newcommand{\categoriedeglossemes}{\categorie{_\star g}}
\newcommand{\glosseme}{{_\star g}}
\newcommand{\nexus}{_\star n}
\newcommand{\nexuscontenu}{\nu}
\newcommand{\nexusexpression}{n}
\newcommand{\pseudonexus}{_\star n_0}
\newcommand{\pseudonexuscontenu}{\nu_0}
\newcommand{\pseudonexusexpression}{n_0}
\newcommand{\syntagmatique}{\semiotique \et}
\newcommand{\interjection}{\nu_0}
\newcommand{\interne}{i}
\newcommand{\externe}{x}
\newcommand{\paradigmatique}{\semiotique \ou}
\newcommand{\langue}{L \paradigmatique}
\newcommand{\texte}{L \syntagmatique}
\newcommand{\semiotiquedenotative}{\interne \semiotique}
\newcommand{\semiotiqueconnotative}{\externe \semiotique}
\newcommand{\semiologie}{_2 \semiotique}
\newcommand{\semiologieinterne}{\interne_2 \semiotique}
\newcommand{\semiologieexterne}{\externe_2 \semiotique}
\newcommand{\metasemiologie}{_3 \semiotique}
\newcommand{\metasemiologieinterne}{\interne_3 \semiotique}
\newcommand{\metasemiologieexterne}{\externe_3 \semiotique}
\newcommand{\plerematique}{\boldsymbol\Gamma}
\newcommand{\cenematique}{\boldsymbol G}
\newcommand{\paradigme}[1]{\lt #1 \gt}
\newcommand{\op}{Op}
\newcommand{\operation}{\op}
\newcommand{\substitution}{\bsemi}
\newcommand{\commutation}{;}
\newcommand{\variante}{var.}
\newcommand{\var}{var.}
\newcommand{\variantede}[1]{\variante(#1)}
\newcommand{\varietede}[1]{\variete(#1)}
\newcommand{\presyntagmatique}{\pre\syntagmatique}
\newcommand{\ligne}{^{\#}\odot}
\newcommand{\lignecontenu}{\gamma\ligne}
\newcommand{\ligneexpression}{g\ligne}
\newcommand{\preligne}{\pre \ligne}
\newcommand{\prelignecontenu}{\pre \lignecontenu}
\newcommand{\preligneexpression}{\pre \ligneexpression}
\newcommand{\cote}{_{\#}\odot}
\newcommand{\cotecontenu}{\gamma\cote}
\newcommand{\coteexpression}{g\cote}
\newcommand{\pleremateme}{\gamma}
\newcommand{\cenemateme}{g}
\newcommand{\denotatif}{\externe \cenemateme}
\newcommand{\connotatif}{\externe \pleremateme}
\newcommand{\determinant}{\ggg \moveleft 3pt \rightarrow}
\newcommand{\determine}{ \leftarrow \moveleft 3pt \lll}
\newcommand{\interdependant}{\leftrightarrow}
\newcommand{\constellatif}{\arrowvert}
\newcommand{\constellation}{\arrowvert}
\newcommand{\element}{_\star l}
\newcommand{\elements}{_\star ll}
\newcommand{\elementcontenu}{\lambda}
\newcommand{\elementscontenu}{\lambda \lambda}
\newcommand{\elementexpression}{l}
\newcommand{\elementsexpression}{ll}
\newcommand{\nexie}{_\star nII}
\newcommand{\nexiecontenu}{\nu II}
\newcommand{\nexieexpression}{nII}
\newcommand{\pseudonexie}{_\star nII_0}
\newcommand{\pseudonexiecontenu}{\nu II_0}
\newcommand{\pseudonexieexpression}{nII_0}
\newcommand{\syntagmatie}{S}
\newcommand{\syntagmatiecontenu}{\Sigma}
\newcommand{\reduction}{\equiv}
\newcommand{\transposition}{\underline{ \eqsim}}
\newcommand{\taxeme}{_\star G}
\newcommand{\connotateur}{x \Gamma}
\newcommand{\continuer}{\lt}
\newcommand{\continue}{\gt}
\newcommand{\sommemaximale}{\odot}
\newcommand{\taxemedirectif}{_\star D}
\newcommand{\directif}{_\star D}
\newcommand{\taxemeconstitutif}{_\star M_{\prime}}
\newcommand{\constitutif}{_\star M_{\prime}}
\newcommand{\taxemeflexif}{_\star P_{\prime}}
\newcommand{\flexif}{_\star P_{\prime}}
\newcommand{\flexifsimple}{_\star P}
\newcommand{\thematif}{_\star P_t}
\newcommand{\categoriedetaxemes}{\categorie{\taxeme}}
\newcommand{\categorietaxemes}{\categoriedetaxemes}
\newcommand{\taxemefondamental}{_\star P_\curvearrowright}
\newcommand{\taxemeconverti}{_\star P_\curvearrowleft}
\newcommand{\taxemesemifondamental}{_\star P_{\curvearrowleft\curvearrowright}}
\newcommand{\taxemeambifondamental}{_\star P_{\curvearrowright\curvearrowleft}}
\newcommand{\varietefondamentale}{_\star P_{\curvearrowright\curvearrowleft\curvearrowright}}
\newcommand{\varietesemifondamentale}{_\star P_{\curvearrowright\curvearrowleft\curvearrowleft\curvearrowright}}
\newcommand{\varieteconvertie}{_\star P_{\curvearrowright\curvearrowleft\curvearrowleft}}
\newcommand{\varietedirection}{_\star P_d}
\newcommand{\varietededirectionthematif}{_\star P_d}
\newcommand{\varieteflexion}{_\star P_\tau}
\newcommand{\varietedeflexionthematif}{_\star P_\tau}
\newcommand{\thematifthematise}{_\star P_\vartheta}
\newcommand{\unitehomogene}{{}^{\unicode{5777} /}}
\newcommand{\succession}{\unitehomogene}
\newcommand{\categoriehomogene}{{}_{\unicode{450}/}}
\newcommand{\unitehomosousgenerique}{{}^{\unicode{5777}}}
\newcommand{\categoriehomosousgenerique}{{}_{\unicode{5777}}}
\newcommand{\ensemble}{\unitehomosousgenerique}
\newcommand{\taxememedian}{_\star G_i}
\newcommand{\taxemeperipherique}{_\star G_p}
\newcommand{\taxemesemimedian}{_\star G_{pi}}
\newcommand{\taxemeambimedian}{_\star G_{ip}}
\newcommand{\varietemediane}{_\star G_{ipi}}
\newcommand{\varietesemimediane}{_\star G_{ippi}}
\newcommand{\varieteperipherique}{_\star G_{ipp}}
\newcommand{\taxemecentral}{_\star G_c}
\newcommand{\taxememarginal}{_\star G_m}
\newcommand{\taxemesemicentral}{_\star G_{mc}}
\newcommand{\taxemeambicentral}{_\star G_{cm}}
\newcommand{\glossememedian}{_\star g_i}
\newcommand{\glossemeperipherique}{_\star g_p}
\newcommand{\glossemesemimedian}{_\star g_{pi}}
\newcommand{\glossemeambimedian}{_\star g_{ip}}
\newcommand{\gvarietemediane}{_\star g_{ipi}}
\newcommand{\gvarietesemimediane}{_\star g_{ippi}}
\newcommand{\gvarieteperipherique}{_\star g_{ipp}}
\newcommand{\glossemecentral}{_\star g_c}
\newcommand{\glossememarginal}{_\star g_m}
\newcommand{\glossemesemicentral}{_\star g_{mc}}
\newcommand{\glossemeambicentral}{_\star g_{cm}}
\newcommand{\glossemepremier}{_\star g \prime}
\newcommand{\varietecentrale}{_\star G_{cmc}}
\newcommand{\varietesemicentrale}{_\star G_{cmmc}}
\newcommand{\varietemarginale}{_\star G_{cmm}}
\newcommand{\glossemedirectif}{_\star d}
\newcommand{\gdirectif}{_\star d}
\newcommand{\glossemeconstitutif}{_\star m_{\prime}}
\newcommand{\gconstitutif}{_\star m_{\prime}}
\newcommand{\glossemeflexif}{_\star p_{\prime}}
\newcommand{\gflexif}{_\star p_{\prime}}
\newcommand{\glossemethematif}{_\star p_{t}}
\newcommand{\gthematif}{_\star p_{t}}
\newcommand{\exposantfondamental}{_\star p^\curvearrowright}
\newcommand{\exposantconverti}{_\star p^\curvearrowleft}
\newcommand{\exposantsemifondamental}{_\star p^{\curvearrowleft\curvearrowright}}
\newcommand{\exposantambifondamental}{_\star p^{\curvearrowright\curvearrowleft}}
\newcommand{\gvarietesemifondamentale}{_\star p^{\curvearrowright\curvearrowleft\curvearrowleft\curvearrowright}}
\newcommand{\gvarietefondamentale}{_\star p^{\curvearrowright\curvearrowleft\curvearrowright}}
\newcommand{\gvarieteconvertie}{_\star p^{\curvearrowright\curvearrowleft\curvearrowleft}}
\newcommand{\gthematie}{_\star p}
\newcommand{\thematie}{_\star p}
\newcommand{\gcaractere}{_\star q}
\newcommand{\gvarietecentrale}{_\star g_{cmc}}
\newcommand{\gvarietesemicentrale}{_\star g_{cmmc}}
\newcommand{\gvarietemarginale}{_\star g_{cmm}}
\newcommand{\symphtongue}{_\star f}
\newcommand{\autophtongue}{_\star r}
\newcommand{\autopleremateme}{\rho}
\newcommand{\sympleremateme}{\phi}
\newcommand{\autocenemateme}{r}
\newcommand{\syncenemateme}{f}
\newcommand{\constitutifsimple}{_\star M}
\newcommand{\constituant}{_\star m}
\newcommand{\exposant}{_\star p}
\newcommand{\formatif}{\Pi_{\prime}}
\newcommand{\formatifsimple}{\Pi}
\newcommand{\prosodie}{P_{\prime}}
\newcommand{\prosodiesimple}{P}
\newcommand{\morphemeintense}{\pi_{n}}
\newcommand{\morphemeextense}{\pi_{\nu}}
\newcommand{\prosodemeintense}{p_{n}}
\newcommand{\prosodemeextense}{p_{\nu}}
\newcommand{\morphemedirectif}{\delta}
\newcommand{\pleremeconstitutif}{\mu_{\prime}}
\newcommand{\morphemeflexif}{\pi_{\prime}}
\newcommand{\plerematemethematif}{\pi_{t}}
\newcommand{\plereme}{\mu}
\newcommand{\morpheme}{\pi}
\newcommand{\prosodemedirectif}{d}
\newcommand{\cenemeconstitutif}{m_{\prime}}
\newcommand{\prosodemeflexif}{p_{\prime}}
\newcommand{\cenematemethematif}{p_{t}}
\newcommand{\ceneme}{m}
\newcommand{\prosodeme}{p}
\newcommand{\catalysepar}{\ge}
\newcommand{\implique}{\subset}
\newcommand{\impliquepar}{\supset}
\newcommand{\caractere}{_\nu}
\newcommand{\caractereintense}{_\star C_n}
\newcommand{\caractereextense}{_\star C_\nu}
\newcommand{\derivatif}{\Phi}
\newcommand{\radical}{P}
\newcommand{\caracterenominal}{K_n}
\newcommand{\caractereverbal}{K_\nu}
\newcommand{\consonne}{F}
\newcommand{\voyelle}{R}
\newcommand{\accent}{C_n}
\newcommand{\modulation}{C_\nu}
\newcommand{\taxemeprimaire}{_\star G_1}
\newcommand{\taxemesecondaire}{_\star G_2}
\newcommand{\taxemesemiprimaire}{_\star G_{21}}
\newcommand{\taxemeambiprimaire}{_\star G_{12}}
\newcommand{\varieteprimaire}{_\star G_{121}}
\newcommand{\varietesemiprimaire}{_\star G_{1221}}
\newcommand{\varietesecondaire}{_\star G_{122}}
\newcommand{\taxemeprincipal}{_\star G_I}
\newcommand{\taxemeaccessoire}{_\star G_{II}}
\newcommand{\taxemesemiprincipal}{_\star G_{s}}
\newcommand{\taxemeambiprincipal}{_\star G_{a}}
\newcommand{\varieteprincipale}{_\star G_{aI}}
\newcommand{\varietesemiprincipale}{_\star G_{as}}
\newcommand{\varieteaccessoire}{_\star G_{aII}}
\newcommand{\glossemeprimaire}{_\star g_1}
\newcommand{\glossemesecondaire}{_\star g_2}
\newcommand{\glossemesemiprimaire}{_\star g_{21}}
\newcommand{\glossemeambiprimaire}{_\star g_{12}}
\newcommand{\gvarieteprimaire}{_\star g_{121}}
\newcommand{\gvarietesemiprimaire}{_\star g_{1221}}
\newcommand{\gvarietesecondaire}{_\star g_{122}}
\newcommand{\glossemeprincipal}{_\star g_I}
\newcommand{\glossemeaccessoire}{_\star g_{II}}
\newcommand{\glossemesemiprincipal}{_\star g_{s}}
\newcommand{\glossemeambiprincipal}{_\star g_{a}}
\newcommand{\gvarieteprincipale}{_\star g_{aI}}
\newcommand{\gvarietesemiprincipale}{_\star g_{as}}
\newcommand{\gvarieteaccessoire}{_\star g_{aII}}
\newcommand{\unitemonoplane}{^{\glosseme}}
\newcommand{\monoplan}{\unitemonoplane}
\newcommand{\signe}{^{\gamma g}}
\newcommand{\uniteintrinseque}{\smile}
\newcommand{\unitenonintrinseque}{\frown}
\newcommand{\glossematieintrinseque}{\asymp}
\newcommand{\categorieintrinseque}[1]{>#1<}
\newcommand{\glossie}[1]{)#1(}
\newcommand{\cenematieintrinseque}{g^\glossematieintrinseque}
\newcommand{\cenematienonintrinseque}{g^\unitenonintrinseque}
\newcommand{\uniteheterogene}[1]{#1^\#}
\newcommand{\categorieheterogene}[1]{#1_\#}
\newcommand{\categorieheterosousgenerique}[1]{#1_{\#\prime}}
\newcommand{\uniteheterotypique}{^{\#\prime}}
\newcommand{\categorieheterotypique}[1]{#1_{\#\prime}}
\newcommand{\uniteheterosousgenerique}{\syntagme}
\newcommand{\syntagme}{_\star s}
\newcommand{\syntagmecontenu}{\sigma}
\newcommand{\syntagmeexpression}{s}
\newcommand{\textdoublebarpipe}{\unicode{450}}
\newcommand{\unitehomotypique}{^{\times\prime}}
\newcommand{\categoriehomotypique}[1]{#1_{\times\prime}}
\newcommand{\uniteheterosoustypique}{^\textdoublebarpipe}
\newcommand{\categorieheterosoustypique}[1]{#1_\textdoublebarpipe}
\newcommand{\unitehomosoustypique}{^\times}
\newcommand{\categoriehomosoustypique}[1]{#1_\times}
\newcommand{\suite}{\uniteheterosoustypique}
\newcommand{\groupe}{\unitehomosoustypique}
\newcommand{\uniteheterotagmatique}{^{\neq}}
\newcommand{\categorieheterotagmatique}[1]{#1_{\neq}}
\newcommand{\unitehomotagmatique}{^{=}}
\newcommand{\categoriehomotagmatique}[1]{#1_{=}}
\newcommand{\uniteheterosoustagmatique}{^\textdoublebarpipe}
\newcommand{\categorieheterosoustagmatique}[1]{#1_\textdoublebarpipe}
\newcommand{\conglomerat}{\uniteheterotagmatique}
\newcommand{\coomplexe}{\unitehomotagmatique}
\newcommand{\uniteheterosoustagmatique}{^{\neq\prime}}
\newcommand{\categorieheterosoustagmatique}[1]{#1_{\neq\prime}}
\newcommand{\unitehomosoustagmatique}{^{= \prime}}
\newcommand{\categoriehomosoustagmatique}[1]{#1_{= \prime}}
\newcommand{\uniteheterosoustagmatique}{^\textdoublebarpipe}
\newcommand{\categorieheterosoustagmatique}[1]{#1_\textdoublebarpipe}
\newcommand{\uniteminimale}{^{(n)}}
\newcommand{\uniteminimalede}[1]{^{(#1)}}
\newcommand{\Uniteminimale}[1]{{#1}^{(n)}}
\newcommand{\uniteidentite}{^{\substitution}}
\newcommand{\unitedifferentielle}{^{???}}
\newcommand{\Unitedifferentielle}[1]{{#1}^{???}}
\newcommand{\expressionpour}{E}
\newcommand{\permutation}{!}
\newcommand{\mot}{^{(\gamma g \permutation)}}
\newcommand{\racine}{\sqrt{}}
\newcommand{\affixe}{a}
\newcommand{\steme}{t}
\newcommand{\formant}{fm}
\newcommand{\alternative}{:}
\newcommand{\taxie}{\Unite{\taxeme}}
\newcommand{\intense}{_n}
\newcommand{\extense}{_\nu}
\newcommand{\caracteristique}{_\star q^S}
\newcommand{\caracteristiquecontenu}{۹^S}
\newcommand{\caracteristiqueexpression}{q^S}
\newcommand{\caracteristiqueintense}{\caracteristique \intense}
\newcommand{\caracteristiqueintensecontenu}{\caracteristiquecontenu \intense}
\newcommand{\caracteristiqueintenseexpression}{\caracteristiqueexpression \intense}
\newcommand{\caracteristiqueextense}{\caracteristique \extense}
\newcommand{\caracteristiqueextenseexpression}{\caracteristiqueexpression \extense}
\newcommand{\caracteristiquenominale}{۹^S \intense}
\newcommand{\caracteristiqueverbale}{۹^S \extense}
\newcommand{\pseudocaracteristique}{_\star q_0}
\newcommand{\pseudocaracteristiquecontenu}{۹_0}
\newcommand{\pseudocaracteristiqueexpression}{q_0}
\newcommand{\theme}{_\star \unicode{421}^S}
\newcommand{\themecontenu}{ϑ^S}
\newcommand{\themeexpression}{\unicode{421}^S}
\newcommand{\themeintense}{\theme \intense}
\newcommand{\themeintenseexpression}{\themeexpression \intense}
\newcommand{\themeextense}{\theme \extense}
\newcommand{\themeextensecontenu}{\themecontenu \extense}
\newcommand{\themeextenseexpression}{\themeexpression \extense}
\newcommand{\themenominal}{ϑ^S \intense}
\newcommand{\themesyllabique}{\unicode{421}^S_n}
\newcommand{\pseudotheme}{_\star \unicode{421}_0}
\newcommand{\pseudothemecontenu}{ϑ_0}
\newcommand{\pseudothemeexpression}{\unicode{421}_0}
\newcommand{\pseudosyllabe}{\unicode{421}_0}
\newcommand{\relatif}{\syntagmateme X}
\newcommand{\verbe}{\conjonction(\nexus)}
\newcommand{\compositum}{\syntagme^S}
\newcommand{\juxtaposition}{\syntagme^\unicode{421}}
\newcommand{\conjonction}{_\star \unicode{421}_0X}
\newcommand{\conjonctioncontenu}{ϑ_0X}
\newcommand{\opGg}{\boldsymbol{_\star Gg}}
\newcommand{\opg}{\boldsymbol{_\star g}}
\newcommand{\opgI}{\boldsymbol{_\star gI}}
\newcommand{\opgII}{\boldsymbol{_\star gII}}
\newcommand{\opgIII}{\boldsymbol{_\star gIII}}
\newcommand{\opgIV}{\boldsymbol{_\star gIV}}
\newcommand{\sopg}[1]{\boldsymbol{_\star g #1}}
\newcommand{\opn}{\boldsymbol{_\star n}}
\newcommand{\ops}{\boldsymbol{_\star s}}
\newcommand{\opG}{\boldsymbol{_\star G}}
\newcommand{\opGIII}{\boldsymbol{_\star GIII}}
\newcommand{\sopG}[1]{\boldsymbol{_\star G #1}}
\newcommand{\opt}{\boldsymbol{_\star t}}
\newcommand{\operatoire}[1]{/#1/}
\newcommand{\syntagmateme}{_\star z}
\newcommand{\syntagmatemecontenu}{\zeta}
\newcommand{\syntagmatemeexpression}{z}
\newcommand{\syllabe}{z}
\newcommand{\nom}{\zeta}
\newcommand{\jonction}{\syntagmatie \unitecomplexe}
\newcommand{\jonctioncontenu}{\syntagmatiecontenu \unitecomplexe}
\newcommand{\jonctionexpression}{\syntagmatieexpression \unitecomplexe}
\newcommand{\espece}{_{ᚑ \prime } \odot}
\newcommand{\sousespece}{_ᚑ \odot}
\newcommand{\type}{_{\times \prime} \odot}
\newcommand{\soustype}{_{\times} \odot}
\newcommand{\tagma}{_{=} \odot}
\newcommand{\soustagma}{_{= \prime} \odot}
\newcommand{\sommeminimale}{I}
\newcommand{\Ufonction}{\fonction}
\newcommand{\Ufonctif}{F}
\newcommand{\Uchaine}{\Ufonctif^N}
\newcommand{\Uunite}{\Ufonctif^n}
\newcommand{\Uidentique}{\equiv}
\newcommand{\Uparadigme}[1]{\lt #1 \gt}
\newcommand{\Usomme}[1]{\Uparadigme{#1}^+}
\newcommand{\Ucategorie}[1]{\categorie{#1}}
\newcommand{\Ucategorieexhaustive}[1]{\categorie{#1}^+}
\newcommand{\Uderivation}{\lhd}
\newcommand{\UDerivation}{\rhd}
\newcommand{\avoir}{???}
$
Definiendum
n°
Definiens
sélection
27
sélection est une relation entre une constante et une variable .
solidarité
58
Une solidarité est une relation entre deux constantes . Quand il n'y a que deux et seulement deux constantes qui contractent la solidarité, la solidarité peut être appelée une connexion bilatérale .
combinaison
59
combinaison est une relation entre deux variables .
complémentarité, corrélation bilatérale
93
Une complémentarité est une corrélation entre deux constantes . Si les constantes qui contractent la complémentarité sont au nombre de deux et de seulement deux, la complémentarité peut être appelée une corrélation bilatérale .
autonomie
94
Une autonomie est une corrélation entre deux variables .
syncrétisation
110
Une syncrétisation est une solidarité entre une variante et une superposition . La variante est dite syncrétisante relativement au syncrétisme qui est établi par la superposition .
défectivation
111
défectivation est une solidarité entre une variante et une séjonction . La variante est dite défectiver (symbole : $\defectiver$) la catégorie défective , et celle-ci est dite être défectivée par (symbole : $\defectivee$) la variante .
interdépendance
125
Une interdépendance est une fonction entre deux constantes .
constellation
126
Une constellation est une fonction entre deux variables .
déterminant
127
Un déterminant ou fonctif déterminant (symbole :$\determinant$) est la variable dans une détermination .
déterminé
128
Un déterminé ou fonctif déterminé (symbole : $\determine$) est la constante dans une détermination .
interdépendants
129
Des interdépendants (symbole : $\interdependant$) sont des fonctifs qui contractent une interdépendance .
constellatifs
130
Les constellatifs (symbole : $\constellatif$ ) sont des fonctifs qui contractent une constellation .
spécification
135
Une spécification est une corrélation entre une constante et une variable .
cohésion, cohésif
148
Cohésion est un terme commun pour interdépendance et détermination . Des fonctifs qui contractent une cohésion mutuelle sont dits cohésifs . Pour une cohésion paradigmatique on utilise le symbole $\cohesionparadigmatique$, pour une cohésion syntagmatique, le symbole
plan interne, plan de contenu interne, plan d'expression interne, fonctif interne
165
Un plan interne (symbole : $\planinterne$) est une composante d'une sémiotique dénotative .
plan externe, plan de contenu externe, plan d'expression externe, fonctif externe
166
Un plan externe (symbole : $\planexterne$) est une composante d'une sémiotique connotative .
plan sémiologique, plan de contenu sémiologique, fonctif sémiologique, descriptif, descripteur
169
Un plan sémiologique (symbole : $\plansemiologique$) est une composante d'une sémiologie .
plan métasémiologique, plan de contenu métasémiologique, plan d'expression métasémiologique, fonctif métasémiologique, métadescriptif, métadescripteur
170
Un plan métasémiologique (symbole : $\planmetasemiologique$) est une composante d'une métasémiologie .
ligne de contenu et ligne d'expression
190-191
Ligne de contenu (symbole : $\lignecontenu$) et ligne d'expression (symbole : $\ligneexpression$) sont des désignations spéciales attribuées arbitrairement aux lignes dont le nombre dans une syntagmatique est deux et deux seulement.
côté de contenu, côté d'expression
271-272
côté de contenu (symbole : $\cotecontenu$) et côté d'expression (symbole : $\coteexpression$) sont attribuées arbitrairement comme noms distincts pour les côtés dont le nombre dans une paradigmatique est deux et seulement deux.
morphème directif
289
morphème directif (symbole : $\morphemedirectif$) est un glossème directif plérématique .
plérème constitutif
290
plérème constitutif (symbole : $\pleremeconstitutif$) est un glossème constitutif plérématique .
morphème flexif
291
morphème flexif (symbole : $\morphemeflexif$) est un glossème flexif plérématique .
plérématème thématif
292
plérématème thématif (symbole : $\plerematemethematif$) est un glossème thématif plérématique .
plérème
293
Un plérème (symbole : $\plereme$) est un constituant plérématique .
morphème
294
Un morphème (symbole : $\morpheme$) est un exposant plérématique .
prosodème directif
295
prosodème directif (symbole : $\prosodemedirectif$glossème directif cénématique .
cénème constitutif
296
cénéme constitutif (symbole : $\cenemeconstitutif$) est un glossème constitutif cénématique .
prosodème flexif
297
prosodème flexif (symbole : $\prosodemeflexif$) est un glossème flexif cénématique .
cénématème thématif
298
cénématème thématif (symbole : $\cenematemethematif$) est un glossème thématif cénématique .
cénème
299
Un cénème (symbole : $\ceneme$constituant cénématique .
prosodème
300
Un prosodème (symbole : $\prosodeme$) est un exposant cénématique .
syntagme
380
Un syntagme (symbole : $\syntagme$unité hétérosousgénérique .
suite
384
Une suite (symbole : $\suite$) est une unité hétérosoustypique .
groupe
386
Un groupe (symbole : $\groupe$) est une unité homosoustypique .
conglomérat
388
conglomérat (symbole : $\conglomerat$) est une unité hétérotagmatique .
cómplexe
390
cómplexe (symbole : $\coomplexe$) est une unité homotagmatique .
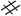 .
.
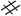 .
.